Thomson's variational measure
Real Analysis Exchange 24 (1998/9), No. 2, 523-566.
Mathematical reviews subject classification: 26A45, 26A46, 26A24.
Abstract
For a local system and a function f:R → R, Thomson defines (see B. S. Thomson, Real functions, Lect. Notes in Math., 1170, Springer-Verlag, 1985) the metric outer measure
and a function f:R → R, Thomson defines (see B. S. Thomson, Real functions, Lect. Notes in Math., 1170, Springer-Verlag, 1985) the metric outer measure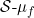 on R.
on R.
We introduced in Real Functions - Current Topics the properties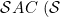 -absolute continuity) and
-absolute continuity) and 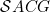 .
.
In this paper we show that for some particular local systems, such as 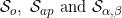 , there is a strong relationship between
, there is a strong relationship between 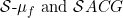 .
.
For example (see Theorem 8.4):
A function f:[a,b] → R is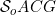 on a Lebesgue measurable set E \subset [a,b]
on a Lebesgue measurable set E \subset [a,b]
⇒ 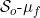 is absolutely continuous on E
is absolutely continuous on E
⇒ f is VB*G ∩ (N) on E and f is continuous at each point of E
In Theorem 5.1, we show that:
A function f:[a,b]→ R, Lebesgue measurable on E ⊆ [a,b], is on E if and only if
on E if and only if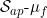 is absolutely continuous on E.
is absolutely continuous on E.
This result remains true if is replaced by
is replaced by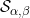 with
with![\small\alpha ,\beta \in \bigl(1/2\,,\,1\bigr] \small\alpha ,\beta \in \bigl(1/2\,,\,1\bigr]](http://latex.codecogs.com/gif.latex?%5Csmall%5Calpha%20%2C%5Cbeta%20%5Cin%20%5Cbigl%281%2F2%5C%2C%2C%5C%2C1%5Cbigr%5D) (see Theorem 5.1).
(see Theorem 5.1).
We also give several characterizations of the VB*G functions on an arbitrary set, that are continuous at each point of that set (see Theorem 7.4).